

计算民盟细胞生命的RTC的备份操作-Calculating
新品快讯
29人已加入
描述
Abstract: Several Dallas Semiconductor real-time clocks (RTCs) integrate a voltage-regulated trickle charger, suitable for charging a manganese-dioxide lithium (ML) coin cell. The depth of discharge during use affects the service life of a ML cell. This application note discusses how to calculate the approximate cell life, based upon cell capacity, load, and depth of discharge.
ML secondary (rechargeable) cells are a relatively new technology, with some important advantages over primary (rechargeable) lithium coin cells (typically CR and BR). Some ML cells are capable of withstanding standard IR reflow profiles, while primary lithium batteries cannot. Using an ML cell can simplify the manufacturing process, eliminating the need to place the coin cell on a PCB post-reflow. A primary cell must either be sized to provide backup power over the expected life of the product, or provisions must be made for the cell to be replaced. While an ML cell has a lower energy density than a primary lithium cell, the ML cell can, in some applications, have smaller capacity, because it is recharged between uses. This allows for a smaller cell, decreasing the required PCB space. An advantage of the ML cell is its relatively low self-discharge rate (compared to capacitors, NiMH, and NiCad batteries) of approximately 1% per year, which allows extended life when the current load is small.
ML cells require a regulated-voltage charging source. Dallas Semiconductor has integrated the required charging source into a family of RTCs. The stand-alone DS12R885 has the charger on chip. In addition, there are module products that include the battery, charger, and RTC in a BGA package.
One issue with secondary cells is the number of charge/discharge cycles that they can withstand during a normal service life. For ML cells, the number of charging cycles is inversely related to the depth of discharge (DoD).
Figure 1 shows the effect that depth of discharge has on the number of charging cycles. The data are taken from the manufacturer of an ML614R battery. We can approximate the number of cycles for a given depth of discharge, and then calculate the approximate time to a particular discharge depth by multiplying the depth of discharge by the nominal cell capacity, and dividing by the load current. From there we can approximate the total life during backup (service life) by multiplying the calculated time by the number of expected cycles: total life = %DoD*(capacity/load)*cycles.
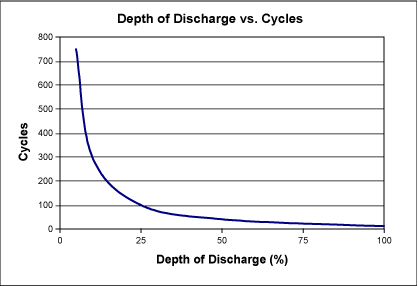
Figure 1. Depth of discharge vs. number of cycles for an ML614R.
We can plot the curves in Figure 2 using 2.2mAH as the battery capacity and using several load values.
An on-line calculator is available to calculate approximate service life based upon user-supplied battery capacity, load, and depth of discharge.
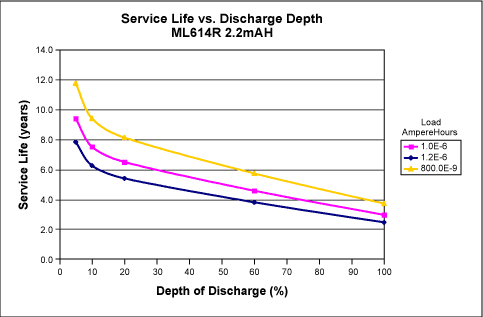
Figure 2. Service life vs. discharge depth for a 22mAH ML614R.
打开APP阅读更多精彩内容
ML secondary (rechargeable) cells are a relatively new technology, with some important advantages over primary (rechargeable) lithium coin cells (typically CR and BR). Some ML cells are capable of withstanding standard IR reflow profiles, while primary lithium batteries cannot. Using an ML cell can simplify the manufacturing process, eliminating the need to place the coin cell on a PCB post-reflow. A primary cell must either be sized to provide backup power over the expected life of the product, or provisions must be made for the cell to be replaced. While an ML cell has a lower energy density than a primary lithium cell, the ML cell can, in some applications, have smaller capacity, because it is recharged between uses. This allows for a smaller cell, decreasing the required PCB space. An advantage of the ML cell is its relatively low self-discharge rate (compared to capacitors, NiMH, and NiCad batteries) of approximately 1% per year, which allows extended life when the current load is small.
ML cells require a regulated-voltage charging source. Dallas Semiconductor has integrated the required charging source into a family of RTCs. The stand-alone DS12R885 has the charger on chip. In addition, there are module products that include the battery, charger, and RTC in a BGA package.
One issue with secondary cells is the number of charge/discharge cycles that they can withstand during a normal service life. For ML cells, the number of charging cycles is inversely related to the depth of discharge (DoD).
Figure 1 shows the effect that depth of discharge has on the number of charging cycles. The data are taken from the manufacturer of an ML614R battery. We can approximate the number of cycles for a given depth of discharge, and then calculate the approximate time to a particular discharge depth by multiplying the depth of discharge by the nominal cell capacity, and dividing by the load current. From there we can approximate the total life during backup (service life) by multiplying the calculated time by the number of expected cycles: total life = %DoD*(capacity/load)*cycles.

Figure 1. Depth of discharge vs. number of cycles for an ML614R.
We can plot the curves in Figure 2 using 2.2mAH as the battery capacity and using several load values.
An on-line calculator is available to calculate approximate service life based upon user-supplied battery capacity, load, and depth of discharge.

Figure 2. Service life vs. discharge depth for a 22mAH ML614R.
Conclusion
ML cells provide additional flexibility for the system designer. The ability to reflow the battery, a smaller footprint, and improved self-discharge characteristics help to reduce product cost and complexity.
声明:本文内容及配图由入驻作者撰写或者入驻合作网站授权转载。文章观点仅代表作者本人,不代表电子发烧友网立场。文章及其配图仅供工程师学习之用,如有内容侵权或者其他违规问题,请联系本站处理。
举报投诉
- 相关推荐
- RTC
-
请问STM32CubeMX 4.20.1自动生成的RTC备份寄存器能否让用户选择?2024-05-06 0
-
生命游戏编程程序分享2014-12-06 0
-
内蒙古银宏干细胞库“生命之光”创新工作室事迹2018-08-15 0
-
PSOC Creator 3.2 RTC实时时钟组件备份怎么实现?2019-09-04 0
-
实时时钟RTC和备份寄存器BKP特征2021-08-02 0
-
关于RTC备份数据区数据丢失问题的讨论2021-08-09 0
-
为什么STM32 RTC BKP备份数据区数据会丢失呢2021-11-15 0
-
架空裸导线载流量和温度的关系计算(Calculating t2008-05-24 1614
-
LPC2138 RTC使用PCONP时的操作注意点2010-03-08 1369
-
Calculating the Error Budget i2009-01-06 949
-
Calculating Energy Usage: How2009-03-23 754
-
嵌入式学习之RTC时钟2021-10-20 441
-
带备份存储器的独立RTC,带低成本MSP430 MCU应用说明2024-09-14 133
-
使用低内存MSP430™ MCU的带备份存储器的外部RTC2024-10-09 106
-
活细胞的“聚光灯”——前沿活细胞成像的案例分享2024-10-24 369
全部0条评论
快来发表一下你的评论吧 !

